Inter Quartile Range (IQR)
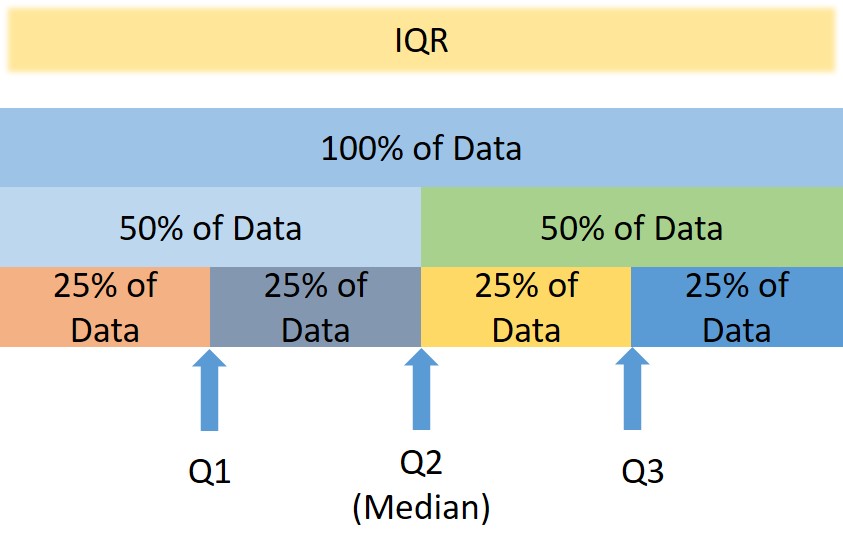
IQR helps to understand spread of data, by dividing the data in to 4 pieces (also known as quarters)
Example:
Imagine there are some set of numbers give, we wanted to understand the data spread, obviously we take average, but average is sensitive to the max and min values, in such case we use IQR for better understanding of data
How to calculate IQR?
- Order the set of numbers in ascending order
- Split the data in to 2 equal halves, it is same as median. We call this as second quartile (Q2), this represents middle value of whole data
- Now let’s take, first equally divided sequence of numbers and find the middle value again, the middle values obtained from first half of numbers is called as first Quartile (Q1) this represents 25% of the data
- Similarly if we find middle number of remaining piece, we call it as third quartile(Q3)
- Now if we compare the difference between Q1, Q2, Q3 we can understand how data is spread out
Advantages of IQR
- We can find the outliers by calculating the Lower fence and Upper fence